
main content:
1. Thermodynamics of the ternary Li-M-O system
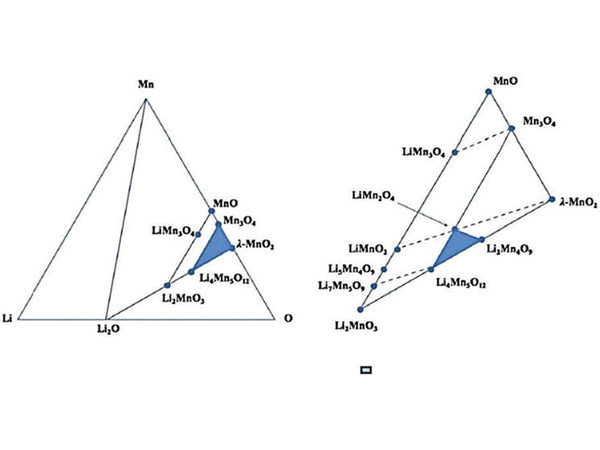
In the actual ternary Li-M-O (M is a transition metal) system, the crystal phase change of the cathode material is more complicated. By comparing the observed OCV values, the following properties can be drawn.
As shown in Table 1, for different transition metal oxides, the contribution of the stability energy is in the range of 0.3 to 0.8V. As the valence of the transition metal increases, the contribution of the stability energy increases. Compared with other alkali metal binary oxides, the stability of lithium binary oxides is poor, which is one of the main characteristics of the Li-M-O system.
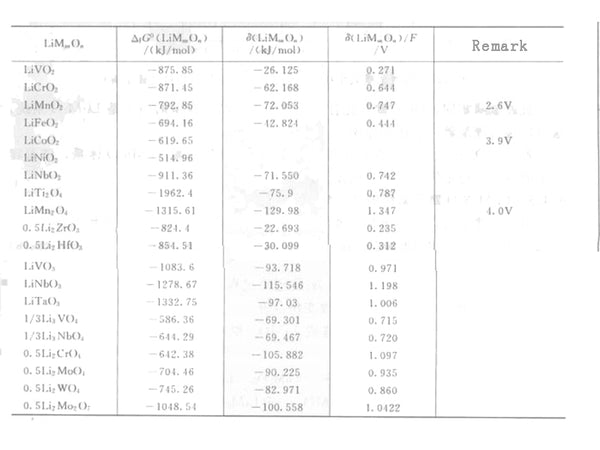
Table 1 Free energy change and stability energy data of formation of lithium transition metal oxide Gibbs at 300K
The redox term can be calculated from the thermodynamic data of Li2O, MOn and MnO2n+1, without any ternary compound information. The calculation results are plotted in Figure 1, and can be compared with a large number of literature values. The redox term can provide a theoretical explanation for the different OCVs of transition metals with different valence states. In the LiMO2 electrode, when M changes from Ti to Co, the transition metal changes from tetravalent to trivalent, and its equilibrium oxygen partial pressure and OCV increase.
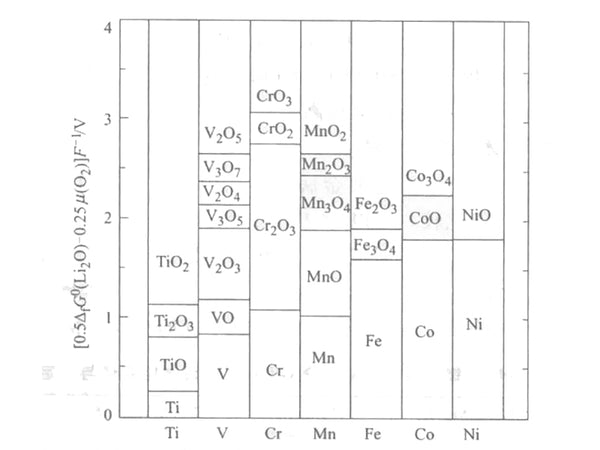
Figure 1 The redox equilibrium potential of transition metal oxides (relative to transition metals)
For the same transition metal, OCV increases with the increase of its valence. A typical example is the Li-M-O system, which can reasonably explain the redox term.
If there are multiple Li-M-O binary oxides in the LiMOn pseudo-binary system, such as the Li-Mn-O system, as the lithium content decreases, the contribution to the stability energy will be more significant.
2. Thermodynamics of Li-M-X-O quaternary system
The cathode reaction of replacing oxide cathode materials with lithium transition metal oxyacid salts can be expressed as follows:
Li+(electrolyte)+e(electrode)+M(XOm)k=MLi(XOm)k——(1)
Transition metal M is a redox element, and its valence changes with the insertion/extraction of lithium. XOm is the main oxyacid radical. It is VO4 in the LiNiVO4 electrode and SO4 in the (FeLi)2(SO4)3 electrode. The electrode reaction is as follows:
Li+(electrolyte)+e(electrode)+NiVO4=LiNiVO4 ——(2)
Li+(electrolyte)+e(electrode)+0.5Fe2(SO4)3=0.5(FeLi)2(SO4)3 ——(3)
the lithium chemical potential of equation (1) can be expressed as:
μ(Li)-μ0(Li)=△fG0{LiM(XOm)k}-△fG0{M(XOm)k}
=△fG0{Li(XOm)n}-△fG0{M(XOm)k}-△fG0{M(XOm)kn}+△fG0{LiM(XOm)k}-[△fG0{Li(XOm)n }+△fG0{M(XOm)k-n}] ——(4)
Using the same concept in the oxide system, the above equation can be written as:
μ(Li)-μ0(Li)={μ(Li)-μ0(Li)}redox+δxom{(LiM)(XOm)k} ——(5)
{μ(Li)-μ0(Li)}redox=△fG0{Li(XOm)n}-[△fG0{M(XOm)k}-△fG0{M(XOm)k-n}
=△[Li0;Li+]+δ{Li(XOm)n}-△[M(2n-1)+;M2n+]-[δ{M(XOm)k}-δ{M(XOm)k-n}] ——(6)
δxom{(LiM)(XOm)k}=△fG0{MLi(XOm)k}-[△fG0{Li(XOm)n}-△fG0{M(XOm)k-n} ——(7)
The first term {μ(Li)-μ0(Li)}redox originates from the difference in valence stability between Li and M in the oxygen-containing salt lattice, and the second term δXOm{(LiM)(XOm)k} is the main body The interaction term between the lithium ion and the M ion in the substance XOm has a fixed valence. It is expected that this value is small and does not depend on the redox element.
In the chemical potential diagram of the quasi-ternary Li-M-XOm system, these two terms can be expressed in a similar way to the ternary system, as shown in Figure 2. The properties and characteristics that are different from the ternary system are summarized as follows.
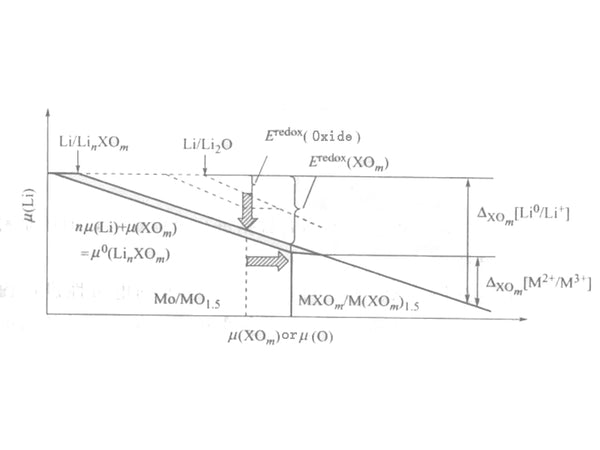
Figure 2 Electrode potential of Li-M-XO system (solid line) and Li-M-O system (dashed line)
The value of the interaction term δXOm{(LiM)(XOm)k} is much smaller than the value of δ{LiMOn} in the ternary system. This is because the valence of M is usually +2, so the mixing of Li+ and M2+ will not cause drastic changes in electrostatic energy. As described below, before mixing, it has greater stability when the oxidized salt is formed.
The change of the redox term is affected by two aspects, one is the stabilization energy of δ{Li(XOm)n}, and the other is that the transition metals in the oxo acid salt are in different valence states and have different stabilization energies. The resulting redox equilibrium migration, δ{M(XOm)k}-δ{M(XOm)k-n}.
The introduction of the foreign element X can obtain a higher working voltage, and the element X should have a higher reactivity with Li2O and can generate stable binary oxides. Li2O is a basic oxide, and acidic oxides can react with Li2O to form stable binary oxides. In order to obtain greater redox equilibrium migration, a stable low-valence crystal structure should be maintained.
As shown in Table 1 and Table 2, the previous item can be calculated by numbers and can be compared in a variety of systems. However, the thermodynamic data for calculating the second term is insufficient, and only part of the data is given in the table. Table 2 lists some data of Fe2+/Fe3+ system.
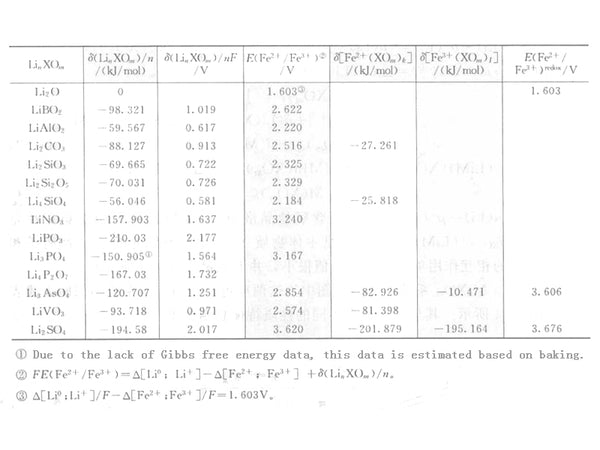
Table 2 The stabilization energy of lithium-metal oxides and the estimated potential of Fe2+/Fe3+ oxidation-reduction
Due to the lack of thermodynamic data, it is difficult to make a comprehensive comparison with the observed data, but the observed data can still be used to explain some phenomena.
For the Nasicon type Fe2(XO4)3, the experimental value can be compared with the electronegativity, and some internal correlations can be found, such as the redox potential of Li0/Li+ and Fe2+/Fe3+ and the thermodynamic data given above satisfy the following Relationship.
FE(Li)redox=△xom[Li0;Li+]-△xom[Mn++;M(n+1)+] ——(8)
△xom[Li0;Li+]=△fG0{Li(XOm)n}-△fG0(Li)-n△fG0(XOm) ——(9)
△xom[Mn++;M(n+1)+]=△fG0{M(XOm)k}-△fG0{M(XOm)k-n}-n△fG0(XOm) ——(10)
The Fe2(SO4)3 value calculated by the above method is 3.676V, which is consistent with the actual value of 3.6V. This is mainly due to the stable energy in equation (6), namely Δ{Li(SO4)1/2} , The migration of redox equilibrium is small. Although the calculated value of oxidation-reduction equilibrium (2.854V) in the non-migration state is consistent with the measured value (2.9V), the potential (3.606V) of Fe2(AsO4)3 calculated by this method is much higher than the measured value. Okada et al. reported the values of a series of substances in Nasicon-type compounds such as: S (3.6V), W (3.0V), Mo (3.0V), As (2.9V), P (2.8V), without considering the value of migration They are S (3.62V), W (2.46V), Mo (2.54V), As (2.85V) and P (3.17V). Although the same order of magnitude is maintained, there are still some differences.
For the Co2+/Co3+ redox system, the oxide redox term {Δ[Li0; Li+]-Δ[Co2+/Co3+]} is calculated as 2.23V. In the inverse spinel or olivine structure, it is not considered In the case of migration, the calculated values obtained are LiCoVO3 3.20V and LiCoPO4 4.41V, respectively. Fey et al. and Okada et al. reported as 3.8V and 4.5V, respectively. There is a 0.5V difference between the two.
The above two examples both show that within the range of ±0.5V, a higher anastomosis can be obtained. The change of the oxide system can be calculated by the stability energy of LiXOm and the redox equilibrium migration. When the first term alone cannot explain its general trend well, the role of the migration term must be properly considered.